

Bravais Lattice

Non-Scalar Structural

In crystallography, a Bravais lattice ¹ represents an infinite array of discrete points, constituting the underlying framework for a crystal structure. These points are generated by a set of discrete translation operations, which can be expressed in three dimensional space as follows.

$${\displaystyle \mathbf{R} = n_{1}\mathbf{a}_{1} + n_{2}\mathbf{a}_{2} + n_{3}\mathbf{a}_{3}}$$

where $n_i$ are any integers, and $a_i$ are known as the lattice vectors spanning the lattice in three-dimensional space. The defining characteristic of a Bravais lattice is that, for any choice of position vector $R$, the lattice has to look exactly the same when viewed from any equivalent lattice point.

Information about the Bravais Lattice can be entered within the lattice editor of Materials Designer, when a new material is being created.

Lattice types

Two Bravais lattices are considered equivalent if they have the same symmetry elements. In this sense, there are 14 possible distinct Bravais lattices in three-dimensional space, grouped together into 7 more general symmetry categorizations known as lattice systems. These two categories are tabulated in the image below for reference purposes.

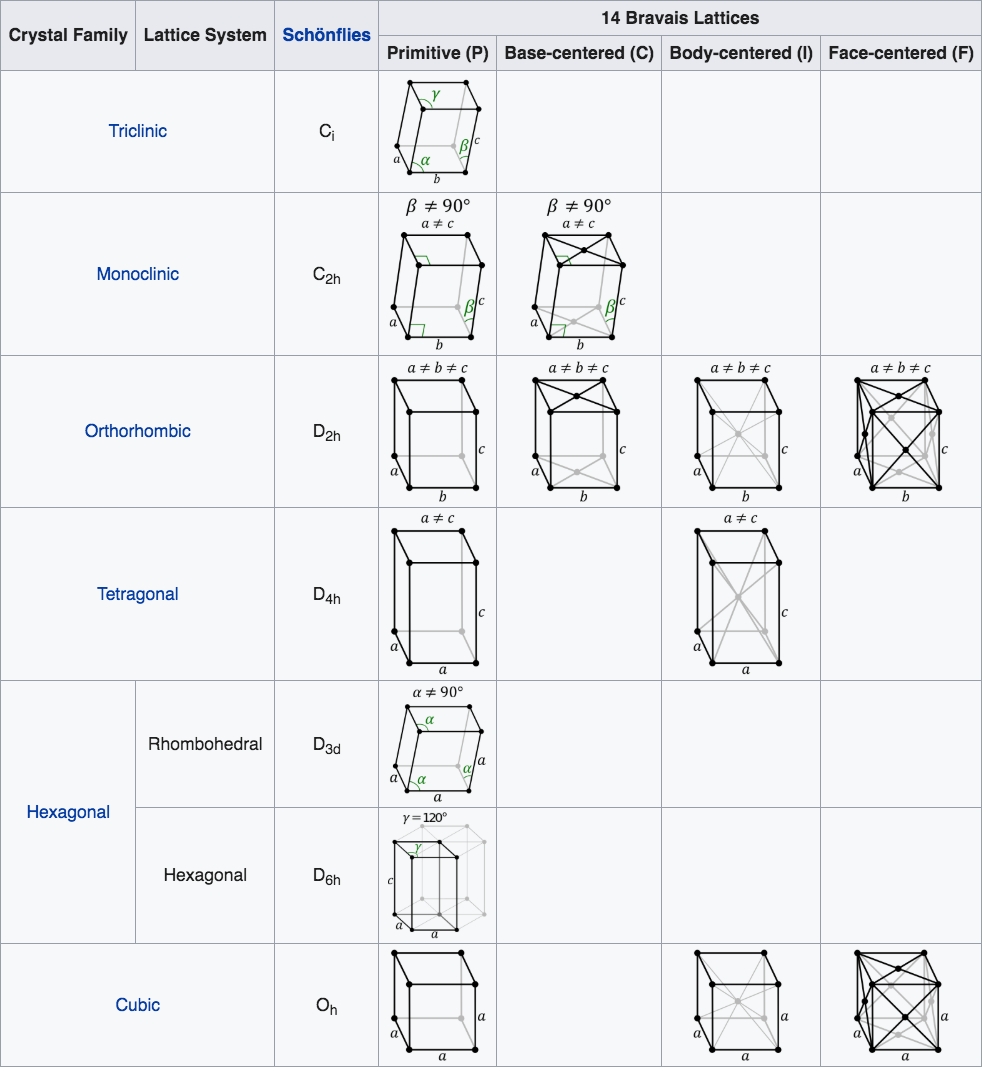
Lattice parameters

Bravais lattices can additionally be described by the lattice parameters defining their unit cells. These parameters comprise information about both the magnitudes of the lattice vectors measuring the lengths of the delimiting axes of the unit cell (the $a$, $b$ and $c$ lattice constants), and the angles between them ($\alpha$, $\beta$ and $\gamma$), as explained in the figure below.

Volume and Density

The corresponding volume $V$ of the above unit cell is consequently given by the scalar triple product between the three lattice vectors defining its axes, as outlined in the formula below. The material density can in addition be obtained by dividing the sum of the atomic masses within the unit cell by this volume.

$$V = \mathbf{a}\cdot(\mathbf{b}\times \mathbf{c})$$

Schema

The JSON schema and an example representation for the properties described in this page can be found for each of the Bravais Lattice, lattice vectors, volume and density.

Links

---

1. Wikipedia Bravais Lattice, Website ↩